A PHILOSOPHY OF THE FOUR-DIMENSIONAL SPACE-TIME
The Worldview of Relative Simultaneity (MURAYAMA Akira)
CHAPTER I
Relativity and Four-dimensional spacetime
[The First half] ---- Outlining the Theory of Relativity ----3. The Relativity of Simultaneity, Time Dilation, and the Length Contraction
(3) The Length Contraction
One of the important logical conclusions of the theory of relativity is the length contraction of other systems of coordinates. This is known both as the FitzGerald-Lorentz contraction and the Lorentz contraction.
Generally speaking, distance can be defined on the basis of the arrival time of light. Distance can be calculated by multiplying the amount of time traveled, by the speed of light. However, a clock runs slowly on the system of coordinates of the other side. For instance, if A travels away at 60% of the speed of light, A's clock gains one second after B's clock (the static system) has gained 1.25 seconds. Accordingly, light travels approximately 300,000 kilometers per second for A (the moving system), whereas light travels 375,000 kilometers per second from the vantage point of B (the static system). That is, the distance of 300,000 kilometers per second for B corresponds to approximately 240,000 kilometers (a reachable distance in 0.8 second for A). (See Figure 1-3-4.)
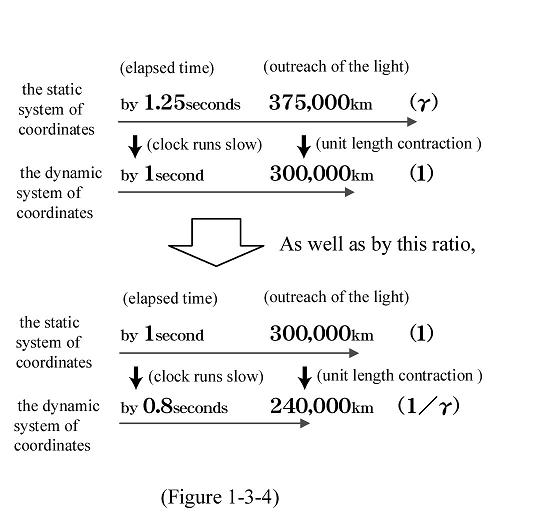
This indicates that the inverse of the degree of time dilation determines the distance contraction on the system of coordinates for the other side. As suggested, if the speed (v) between systems of coordinates is extremely slow as compared with the speed of light (c), the value will show close proximity to the number one; that is, there are only small differences between the scales of both systems of coordinates. Conversely, if the speed between systems of coordinates is appreciably fast enough to be comparable with the speed of light, the value will be less than the number one. That is, the scale on the moving system reflects a larger contraction.
This logic also applies when the moving system and the static system are converted to their opposite positions. More specifically, neither of the two systems of coordinates will unilaterally show an absolute distance contraction, although the distance unit appears to be contracting when judged from the other side's viewpoint.